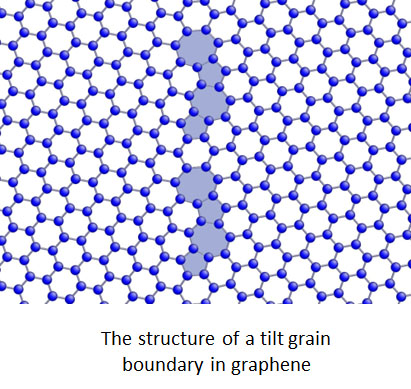
Graphene in its pristine form is one of the strongest materials, but defects influence its strenth. Using atomistic calculations, we find that, counter to standard reasoning, graphene sheets with large-angle tilt boundaries that have a high density of defects are as strong as the pristine material and unexpectedly are much stronger than those with low-angle boundaries having fewer defects. We show that this trend is not explained by continuum fracture models but can be understood by considering the critical bonds in the strained seven-membered carbon rings that lead to failure; the large-angle boundaries are stronger because they are able to better accommodate these strained rings.